The best mathematical tasks are often elegantly simple.  Bells and whistles are not needed and, indeed, often they are distractions from the intended learning. To keep a task focused, thought should be given to the following questions­:

• What do I want the children to learn?
• What might be the ‘bumps in the road’ that could cause difficulty when children learn this?
• What is the most effective way for children to learn this?
• How will I help children navigate the ‘bumps in the road’?

A question I’m asked frequently is: how do we effectively support children who may be falling behind in the lesson at the same time as providing deeper thinking for those who have grasped the concept quickly? 

What follows in this blog is a commentary on a pared back but effective Year 2 task, where children find unknown multiplication facts for fives, using known facts. 

Finding unknown multiplication facts

Well, shouldn’t they just learn their facts?  They will, but learning facts does not mean children are learning about multiplication.  In this case, children are learning about one (or two) lot more and one lot less than any given fact.  We might assume this is easy to learn.  However, those assumptions are often proved wrong by several children in every class.

Children who grasp the increasing and decreasing ‘lots’, of five in this case, learn that we can count in fives beyond the facts usually learned as times tables. They also learn about the relationship between the number of lots, the size of the lot (which stays constant) and the effect on the resultant product.  By learning this, children are dealing with a much more complex structure than addition. Multiplication has a far greater impact on the magnitude of the result than addition. This task also introduces arithmetical laws such as distributive law; think of formal multiplication, for example, where we distribute the multiplication 36 x 4 to be 6 x 4 + 30 x 4.

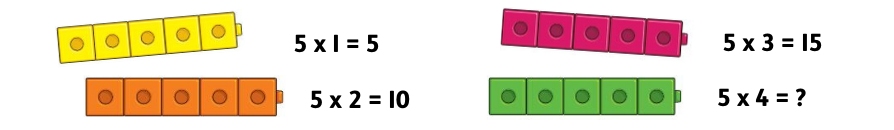
Using patterns of five

Children created a pattern of fives using the five cube trains and to record these numerically.  Simple.

Here is some of what happened. 

T:  Who has worked out twelve lots of five?

C: Sixty.

T: Ok, so twelve lots of five would be sixty.  Does that help you work out thirteen lots of five?

C: Umm…you have sixty, so put that in your head and add on another group. 

T: How many more do you add on?

C: Five more, the groups are five. It’s sixty-five.  

The crucial information is that the task has allowed the child to see that the pattern increased by a group of five more rather than by one more. 

Assessing children’s understanding

Consider how this understanding was assessed in the 2016 SATs paper 3:

Identifying and addressing misunderstanding

As the children were working, the teacher saw a child struggling to record their thinking on a whiteboard.  They had written 5 x 4 + 1 = 30. 

T: What are you trying to work out?

C: Five times five.

T: What do you know so far?

C: Five times four is twenty.

T: And then what’s this one?  (Teacher points to the 5 x 4 +1 in the child’s recording.) Five times four plus one?

C: Twenty-one.

T: Show me four lots of five with your cubes.

C: Five, ten, fifteen, twenty.  (Child counts out 4 trains of 5 cubes.)

(Teacher places a single cube next to the 4 lots of 5.)

T: Is this how we should be adding to find one more group of five?  How many cubes are in each of our groups?

C: Five.

T: So what if instead of adding one more, I add one more lot of five.

(Teacher replaces the single cube with another train of five)

C: Twenty-five.

The child goes on to adjust the number sentence after being asked to make it correct.  The teacher provides a further example for the child to work on. Here the teacher provided a choice between adding a single cube or a train of five cubes. The child chose the train of five cubes and is prompted to say ‘one group’. 

By walking the tables, the teacher was able to intervene responsively and begin to adjust this child’s thinking there and then, so no learning time was lost on repeating the same misconception.

Applying understanding to new scenarios

By this time, some of the children were calculating in fives just beyond one hundred and had clearly grasped the concept.  Children are asked to use what they have found out about fives to write the ten times table.  One child moves the five cube trains on the table to show that two of them are equal to ten each time. 

C: Just use the facts with even numbers.  (Child points at all the ‘even’ multiples of five, 5 x 2 = 10, 5 x 4 =20, 5 x 6 = 30 etc.)

In their recording, they group two five cube trains together each time to demonstrate that there are two fives for every ten. Later, a child is asked about their recording.  Below is what they have noticed.

C: You could say that half of ten is five and half of twenty is ten and half of thirty is fifteen.

T: So if I hid this (teacher covers 5 x 5 = 25), you would know what five times five is?

C: Twenty-five because if you halve fifty you get twenty-five. 

Here the children are discovering common multiples in the 5s and 10s multiplication facts, as well as using the ten times fact to find the five times fact through halving.  This is a strategy that might help them solve something like 50 x 1.26 in the future. 

Though this lesson was elegantly simple, the opportunities that it provided to assess and to deepen and apply understanding are what gave it its power.  Who needs bells, whistles and marching bands anyway?

Rachel Rayner is an adviser in primary mathematics for Herts for Learning and maths consultant for Oxford University Press. She works in primary schools, leads training and works alongside teachers and leaders. Rachel is an author of our new digitally-led primary maths programme, MathsBeat.

References

Transcripts and photos from the MathsBeat Year 2 Teacher’s Handbook.

STA, KS2 Reasoning Paper 3 (2016).