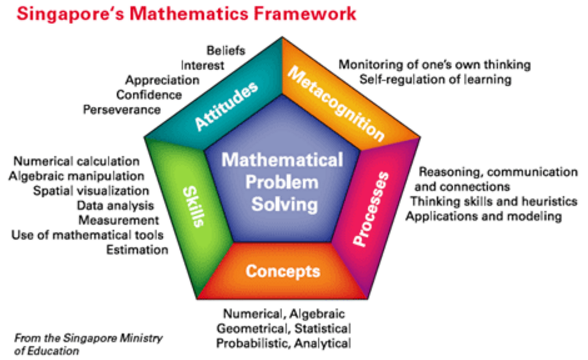
I thought I’d write today about the framework of Singapore’s School Mathematics Curriculum. The framework is captured in a well-known diagram that I’ve attached above, and it provoked a lot of interest among teachers when I was last in the UK in November. This was great to see, because this diagram really is at the heart of the Singapore maths story.
The framework was first devised back in 1990. At first glance, it might seem like any other diagram you might find in any other syllabus document. But believe me: every detail has been carefully thought about and tested over the last 25 or more years.
The quickest way to understand it is to imagine you are building a house. You build a house to have somewhere secure. In just the same way, we want our maths students to acquire a secure grasp of mathematics. How do we know if their maths is secure? If they are confident and capable problem-solvers, that’s how. Once they can problem-solve confidently, they are equipped to use maths throughout their lives and careers. So we put problem-solving at the centre of the ‘house’ – that’s the reason we are building it.
So what’s the first thing every new house needs? Firm foundations of course! Maths concepts build one on top of another. You can only build concepts safely on top if the concepts at the bottom are safely in position. That’s why we don’t rush through the syllabus. This is the core of what has been called the mastery approach. We prefer the term ‘guided discovery’ because we want our students to ‘discover’ the maths for themselves, albeit with guidance. We’ve probably all noticed how much better a student retains knowledge when they’ve figured it out for themselves, than when it’s been learnt by rote.
Next come the walls. These are the skills and processes. I won’t dwell long on the skills: these will be familiar to maths teachers everywhere. The processes that we use in Singapore, though – these have drawn a lot of interest from around the world.
“CPA”, or “Concrete-Pictorial-Abstract” is a term that you may well have heard of, and it is certainly central to our approach. We believe good physical and pictorial models are vital to helping students understand abstract concepts. When teaching ratio, for example, bar models are an excellent way of presenting the information visually. Or when teaching negative numbers, try using algebra discs. The great thing about these is that you can use the physical, ‘Concrete’ discs (they’re normally made of card!), or you can present them as diagrams on the whiteboard (the ‘Pictorial’ form), and then you can progress to the algebraic form. A word of caution: be sparing with how many models you introduce, and make sure you use them consistently thereafter. Students have to invest time at the outset in understanding a new model , so try to make the best possible use of that investment.
There’s so much else that I could say about Processes – in particular the emphasis we place on the precise use of mathematical language and the thought we give to problem-solving heuristics – but space doesn’t allow and we still need to put the roof on the house.
Let’s think about roofs for a moment. A roof is useless without a house underneath it. But equally, a house is useless without a roof to complete it. Reading across to “Attitudes”: a student with bags of confidence and enthusiasm will not get far if they don’t have the knowledge and skills to back that up. Equally, a student with all the learning in the world will not take their maths far if they have no confidence or enthusiasm.
Having the right attitude is crucial and in Singapore we place a lot of emphasis on this part of the framework. We have an advantage because there’s a positive attitude to maths across all of society: teachers, parents and, unsurprisingly, students too. I know this isn’t so straightforward in the UK, but please remember we had to work at this too – Singapore wasn’t born loving maths!
The turnaround starts when maths teachers choose to believe that every child can succeed. This is at the core of the mastery approach and it’s at the core of how we think in Singapore. And it’s supported by the evidence: if you look at the results of the PISA survey, the countries that perform most strongly in maths are also the ones where the children believe that hard work in maths leads to success.
As teachers we need to believe that all our students can succeed. When we believe that, it rubs off onto our students, and success follows. And then, after a few years, you have a generation of parents who believe it too, and a virtuous circle is created.
The second part of the roof, and the last part of the framework, is ‘metacognition’. Quite rightly, as maths teachers, we spend most of our time asking students to do maths. But just occasionally you should try asking them to think about maths. You will be rewarded! Get them to keep a journal – not an exercise book, but somewhere to write down thoughts and reflect on their learning. See if they can explain a new concept in words? Or ask a quicker-learning student to explain a concept to one who hasn’t yet grasped it. Both will benefit.
That’s a whistle-stop tour of the framework. It’s not easy to summarise a quarter of a century’s experience into a blog post. So if you want to find out more, and happen to be coming to the BCME conference this April in Warwick, do come along to my plenary session. I will be talking about the framework in more detail and about what UK maths teachers can adopt or adapt from it. I would like to conclude, though, on this point: the truth is, Singapore learnt a lot from the UK and from UK and US researchers! It was actually by listening, researching and learning from others that we started to build our system into what it is today.
 Berinderjeet Kaur is Professor of Mathematics Education at the National Institute of Education in Singapore. She is the founding chairperson of the Singaporean Mathematics Teachers’ Conference series and a member of the Mathematics Expert Group to PISA 2015. She is passionate about the development of mathematics teachers. Professor Kaur is the Series Consultant for Oxford’s adaptation of Discovering Mathematics to the English national curriculum.
Berinderjeet Kaur is Professor of Mathematics Education at the National Institute of Education in Singapore. She is the founding chairperson of the Singaporean Mathematics Teachers’ Conference series and a member of the Mathematics Expert Group to PISA 2015. She is passionate about the development of mathematics teachers. Professor Kaur is the Series Consultant for Oxford’s adaptation of Discovering Mathematics to the English national curriculum.